Mathematical Musick

The Contrapuntal Formula of Dr. Thomas Campion

By Jeff Lee <godfrey@shipbrook.net>

Thomas Campion (1567-1620) was, among other things, an accomplished musician, lyricist and composer. He was also a musical theoretician; in 1613 he published A New Way of Making Fowre Parts in Counterpoint, a treatise on contrapuntal theory which was republished many times after his death.

In this book, he proposed a novel means of writing four-part music, deriving the upper three parts mathematically from the bass line. In his reasoning, the four parts (of a madrigal, for instance) could be correlated to the four elements: the ground, or bass line, was analogous to the element of Earth; the tenor, to water; the meane, or alto part, was equivalent to the air, and the treble, or soprano part, to the element of fire.

As the upper three parts rested upon the ground, therefore, he concluded that the bass part was the foundation of the entire piece, and that the other three parts naturally proceeded therefrom. His theories were heavily chordal in nature, and in fact (save for passing tones and other ornaments) each of the three upper parts consisted primarily of thirds, fifths and octaves above the ground.

Upon the actual composition of the bass line, Campion gave little direction other than that it should move "not so much by degrees, as by leaps of a third, fourth, or fift, or eight, a sixt being seldome, a seventh never used, and neither of both without the discretion of a skilfull Composer." As the writing of an appropriate bass part is made easier by familiarity with the genuine style, we shall use the grounds of extant chansons and madrigals for our examples, and compare the results of Campion's method with the original four-part arrangements of those madrigals.

Each note of the upper three parts is derived primarily from two components: the interval between the previous note of the part and the previous bass note, and the direction of movement of the bass line.

The first rule Campion gives is this: when determining the direction and distance of the bass line's motion, only intervals of a fourth or less are considered. Intervals of a fifth or more are replaced by their inversions: thus, a bass part which rises a fifth is considered to descend a fourth; a part which rises a sixth is treated as though it descends a third; and a part which rises a seventh is considered to descend a second. Likewise, a part which falls a fifth is considered to rise a fourth, and so on.

To illustrate more clearly: intervals of a second, third or fourth remained unchanged:

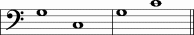
Intervals of a fifth in one direction were treated as a fourth in the other:

rising fifth becomes falling fourth		falling fifth becomes rising fourth

Intervals of a sixth in one direction were treated as a third in the other:

rising sixth becomes falling third		falling sixth becomes rising third

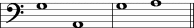
And a seventh in one direction (though cautioned against by Campion) became a second in the other direction:

rising seventh becomes falling second		falling seventh becomes rising second

Intervals greater than an octave were treated as though they fell within the same octave (ninths became seconds, tenths became thirds, et cetera).

The second component of the formula, as mentioned earlier, was the starting distance of each of the three upper parts from the bass. Again, compounded intervals were treated as though they fell in the same octave: so a tenth (being an octave and a third) was treated as a third.

Once the ground has been composed, Campion instructs, one should place the starting notes of the three other parts at thirds, fifths and octaves above the bass note. If the soprano is placed an octave (or two) above the bass, then the alto should begin on a fifth, and the tenor upon a third; if the soprano is placed a third above (or an octave and a third), the alto should be an octave to the bass, and the tenor at a fifth, and so on.

Campion provides us with a table which represents and simplifies the bulk of his theory:

8	3	5
3	5	8

To use the table, find the last note of the part being written, and determine the interval to the bass note (which should be a third, a fifth or an octave). If the bass note moves upwards a second, third or fourth, then find the interval in the bottom row of the chart; if the bass note moves downwards a second, third or fourth, then look in the top row for the interval. (Remember, a part which moves a fifth in one direction is considered to be moving a fourth in the other direction, and so on.)

Once you have found that interval in the chart, then look either above or below it to find the part's new interval from the bass line.

To demonstrate this, we shall use the opening phrase of the bass line from "O occhi, manza mia", a madrigal written by Orlando di Lasso, originally published in Libro de Villanelle, Moresche ed altre canzoni (1581):

First, we need to pick starting notes for our upper three parts. For purposes of comparison with the original piece, we shall use the original starting notes: C in tenor, F in alto, and A in soprano. These notes, fortunately, fall in accordance with Campion's instructions.

The first notes in the bass line does not move, we do not have to move any of the upper parts. However, in such a case, Campion tells us that it is permissible to move the upper three parts to other notes of the same chord, so long as they all move in the same direction; for variety's sake, we shall move the tenor down from C to A.

Next, we see that the bass moves downwards from F to D. We look to see the previous interval, which was a third. Because the bass moves downwards, we look in the top row of the table to find the number 3; looking beneath that numeral we find a 5, indicating that the new tenor note should be a fifth above the bass (in this case, an A).

For the next note, the bass moves upwards to G. As our previous interval was a fifth, we simply look in the bottom row of the table to find a 5, and look above that number to find a 3; therefore, our new tenor note becomes a B-flat, being a third above G.

Next, the bass line moves downwards to F. Our previous interval being a third, we find 3 in the top row of the table, and find 5. As C is the fifth above F, we move the tenor line up to C.

For the next note, the bass descends a fifth — which, as Campion indicated, is equivalent to ascending a fourth. Checking the table against our previous interval of a fifth, we find that the new interval is to be a third above the bass, which in this case is a D.

And finally, the bass ascends a fifth (which, again is converted into a descending fourth), resulting in a tenor note of C. Our tenor line is now complete:

We can now repeat the same operations upon the alto and soprano lines. Again, since the bass line remains upon the same note at the beginning, we can move the other parts within the same chord.

Eventually, we arrive at this result:

Comparing this with di Lasso's original, we find that we have arrived at exactly the same sequences of notes in all three upper parts! Admittedly, this was a fairly simple piece, chosen specifically because the bass sings the root of each chord in the passage, for the rules become more complex when the bass note is not the root (such as when an inverted chord is played or sung, or the bass line consists of passing tones which do not lie in the tonality of the chord).

If the notes arrived at by the formula do not sound pleasing, Campion suggests two methods of sweetening the sound: the first is by adding passing tones in one or more of the upper voices, to avoid an awkward jump; the second is to temporarily reverse the direction in which intervals are derived from the table. In other words, when the bass note descends, rather than finding the previous interval in the top line of the table and looking below it for the new interval, you would find the previous interval in the bottom line, and look above it for the new interval (and vice-versa when the bass note ascends).

Some other rules Campion provides:

• Whenever the bass note is to be the third of the chord (first inversion), use the table normally, but replace a fifth interval with a sixth (for example, if the bass note is E, and the table calls for a fifth in an upper voice part, use a C rather than a B).
• If a voice would move by an augmented second (for example, from E-flat to F-sharp), split the antecedent note into two, and make the latter half jump to the half-step beyond the target note. For example:

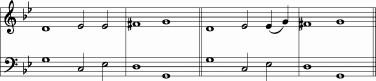
  this	becomes this

• You may insert passing tones into the bass line without refiguring the other parts.
• When the bass note does not lie on the tonic of the chord, it should not be doubled in the upper voices; strengthen the tonic by moving the note that would have doubled the bass onto the root of the chord (which will double another part). When the upper voice moves to its next note, calculate its placement from the note it would have occupied had it not been moved.
• When the bass rises a fifth, third or second, and then immediately falls a fifth or rises a fourth, this indicates a cadence (which Campion calls a 'close'). In such a case, Campion says, it is permissible to create a 4-3 suspension.
• In the 1655 edition published by John Playford, an annotation by Christopher Sympson instructs that whenever the bass falls a fifth, or rises a fourth, whichever part lies a third above the bass in the first of the two chords should always be a major third.
• Sympson also adds that "when any informality doth occur," the composer may use any concordant notes he wishes, so long as he avoids parallel fifths or octaves.
• Finally, Campion provides an example wherein the last chord of a piece is altered "for sweetness sake", such that the treble part plays an octave with the bass, and the third moved to the tenor, as "the eight being a perfect Concord, and better befitting an outward part at the Close". Though he does not specifically instruct it, he also shows the use of the Picardie third, in which the last chord of a minor piece is raised to major.

Also shown by example, but not specifically mentioned in the text, are ornamentations such as suspensions, anticipations, and rhythmic variations amongst the upper voices.

Apart from these rhythmic variations, and the means of avoiding an augmented second (detailed above), Campion's method produces primarily first-species counterpoint (wherein the voices all maintain the same rhythm, and all move at the same time).

To close, let us apply the expanded rules to two more bass lines taken from period examples, and compare them with the originals.

Example 1: Realization of a bass line excerpted from "Si la nature en la diversité", an anonymous chanson published by Pierre Attaingnant, circa 1528.

Below, we have the original realization of the music. Note that our version of the alto line exactly reproduces the original; the tenor was nearly identical, with only a couple of differing notes and some rhythmic variations; and the soprano, while it had the most differences, was nevertheless very close to the original part:

For the final example, we shall use the bass line from the first section of "O Thred of life", a four-part lute song/madrigal by Robert Jones, published in his Fourth Booke of Ayres (1609):

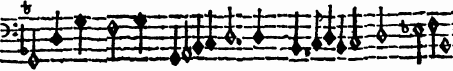
Some basic ornamentation has been added to the notes derived from Campion's formula:

There are definitely more differences in this example, as is to be expected: Jones' contrapuntal style was rather more florid than Campion's, and ornamentation, being more improvisational than formulaic, will definitely introduce an individual character to the piece.

* Misprinted as A in original.

Differences notwithstanding, our version of the piece sounds reasonably good. While it may be surprising that the application of a simple formula can turn a bass line into a four-part madrigal with aesthetically pleasing melodic lines in the three upper parts, it actually does work fairly well. Indeed, it often produces passages nearly (or completely) identical to those created by the original composers, who lacked the benefit of Dr. Campion's "New Way".

---

Bibliography

Playford, John. An Introduction to the Skill of Musick, in two books [...] Second, the Art of Setting or Composing of Musick in Parts, by a most familiar and easie Rule of Counterpoint. Formerly published by Dr Tho. Campion: but now reprinted with large Annotations, By Mr. Christoph. Sympson, and other Additions. London, 1655.

Lagassé, Paul (ed). The Columbia Encyclopedia, Sixth Edition. Columbia University Press, New York, 2001.